이진 탐색 트리

이진 탐색트리란?

이진 탐색트리는 다음과 같은 속성이 있는 자료 구조이다.

• 각 노드에 값이 있고, 각 노드마다 최대 두 개의 자식을 갖는다.
• 왼쪽 자식은 부모보다 키 값이 작고, 오른쪽 자식은 부모보다 키 값이 크다.

이진 탐색트리 예시

• {8,3,10,1,6,14,4,7,13}

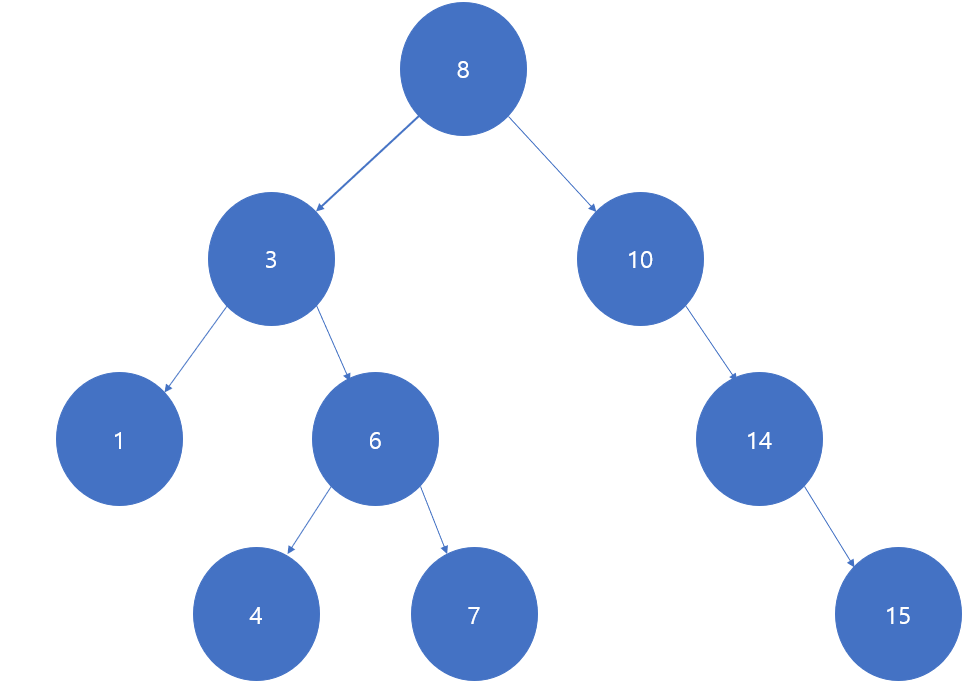
이 노드를 기준으로,

루트 8 의 왼쪽 서브트리는 3부터 그 이하의 노드들로 이루어진 트리이고,

루트 8 의 오른쪽 서브트리는 10부터 그 밑에있는 노드들로 이루어진 트리이다.

 

이진 탐색 트리는 유일하지 않다.

예를 들어 {1, 2, 3, 4}에 대한 이진 탐색 트리는

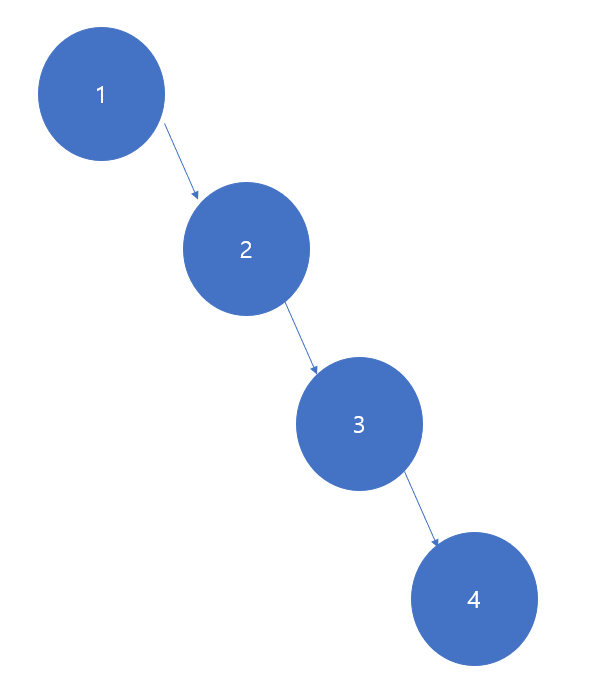
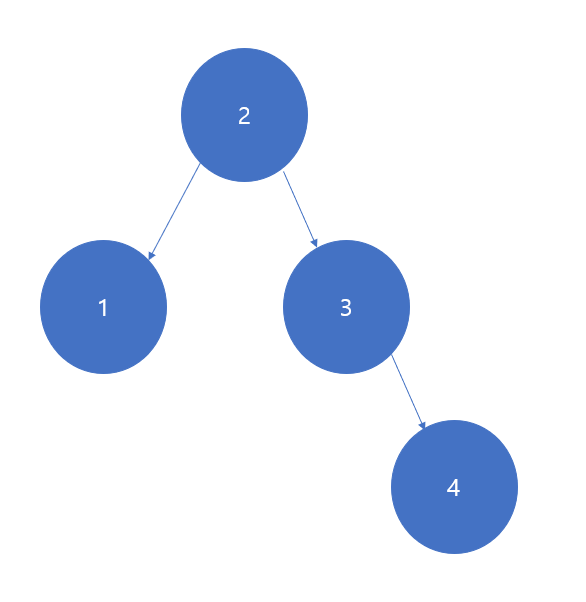
이런식으로 두가지 이상으로 표현 가능하다.

만약 어떤 키 값이 자주 접근될 지 모른다면, 균형잡힌 오른쪽 트리가 유리할 것이고,

만약 1 값이 가장 자주 접근된다면 균형이 잡히지 않은 것이 유리하다

 

• C++ 이진 탐색 트리 구현

//알고리즘 이진 탐색 트리 탐색과 삽입 구현
#include <iostream>
#define null 0
using namespace std;
 
template <typename T>
class Tree;
 
template <typename T>
class Node {
    friend class Tree<T>;
private:
    T key;
    Node* left;
    Node* right;
    Node* parent;
public:
    Node(T key = 0, Node * left = null, Node * right = null, Node* parent = null) {
        this->key = key;
        this->left = left;
        this->right = right;
        this->parent = parent;
    }
    friend ostream& operator<<(ostream& os, const Node<T>& node) {
        os << "[data]" << node.key << endl;
        if (node.left != null) {
            os << "[left]" << node.left->key << endl;
        }
        if (node.right != null) {
            os << "[right]" << node.right->key << endl;
        }
        return os;
    }
};
 
template <typename T>
class Tree {
private:
    Node<T>* root;
public:
    Tree(T key = 0) {
        root = new Node<T>(key);
    }
    //Tree 만들기
    void buildSearchTree() {
        insertNode(new Node<int>(3));
        insertNode(new Node<int>(10));
        insertNode(new Node<int>(14));
        insertNode(new Node<int>(2));
        insertNode(new Node<int>(5));
        insertNode(new Node<int>(11));
        insertNode(new Node<int>(16));
    }
 
    void insertNode(Node<T>* node) {
        //중복이 없을 떄
        if (search(root, node->key) == null) {
            Node<T>* parent = null;
            Node<T>* current = root;
            //작으면 왼쪽, 크면 오른쪽
            //새 노드의 부모가 정해짐
            while (current != null) {
                parent = current;
                if (node->key < parent->key) {
                    current = current->left;
                }
                else {
                    current = current->right;
                }
            }
            if (node->key < parent->key) {
                parent->left = node;
            }
            else {
                parent->right = node;
            }
            cout << "Inserted" << node->key << endl;
        }
    }
    
    Node<T>* getRoot() {
        return root;
    }
    //전위순회
    void preorder(Node<T>* current) {
        if (current != null) {
            visit(current);
            preorder(current->left);
            preorder(current->right);
        }
    }
    
    void visit(Node<T>* current) {
        cout << current->key << " ";
    }
    //탐색 연산
    Node<T>* search(Node<T>* current, T key) {
        if (current == null) return null;
        if (key == current->key) {
            return current;
        }
        else if (key < current->key) {
            search(current->left, key);
        }
        else {
            search(current->right, key);
        }
    }
};
int main()
{
    Tree<int> tree(8);
    tree.buildSearchTree();
    //Tree 출력
    cout << "preorder >>";
    tree.preorder(tree.getRoot());  //루트 값 받아 그 값부터 전위순회
    cout << "\n";
 
    //tree에 값 넣어보기
    tree.insertNode(new Node<int>(4));
    cout << "Preorder >> ";
    tree.preorder(tree.getRoot());
    cout << "\n";
 
    //tree 탐색
    int number;
    cout << "input number to search >>";
    cin >> number;
 
    Node<int>* found = tree.search(tree.getRoot(), number);
 
    if (found != null) {
        cout << "Fount node." << *found << "\n";
    }
    else {
        cout << "Not found node." << "\n";
    }
}
 

 

C++ 이진 탐색 트리 구현하기 (Binary Search Tree)

이 코드는 여기서 참고하였습니다.

 

• 이진 탐색 트리 검색

• 이진 탐색 트리에 원하는 값 k가 들어 있는가?

1. 트리가 비어있다면 k는 트리에 있을 수 없다.

2. 만약 루트의 값이 원하는 값 k라면, 이 값을 리턴한다.

3. 만약 루트의 값이 k보다 크다면 (k가 루트보다 작으면) k는 루트의 왼쪽 서브 트리에 있어야하며,

만약 루트의 값이 k보다 작다면 (k가 루트보다 크다면) k는 루트의 오른쪽 서브 트리에 있어야한다.

4. 2~3의 과정을 반복

 

• 이진 탐색 트리 삭제

• 삭제할 노드가 무엇인가에 따라서 세 가지 경우가 존재한다

1. 노드가 리프이다

-> 그냥 지우자

2. 노드가 하나의 자식을 가진다

-> 노드를 지우고, 자식을 원래 노드의 위치로 옮긴다

3. 노드가 두 자식을 가진다

case1 : 서브 트리의 원소들을 모두 모아 다시 트리를 만든다

case2 : 트리의 모양을 최대한 유지하는 방법.  삭제할 노드의 키 값에서 바로 앞이나 바로 뒤에 있는 노드를 찾고, 이 노드를 원래 노드의 위치로 옮긴 후 다시 옮긴 노드 자리를 두고 1~3 번 과정을 거치는 경우

 

삭제의 예

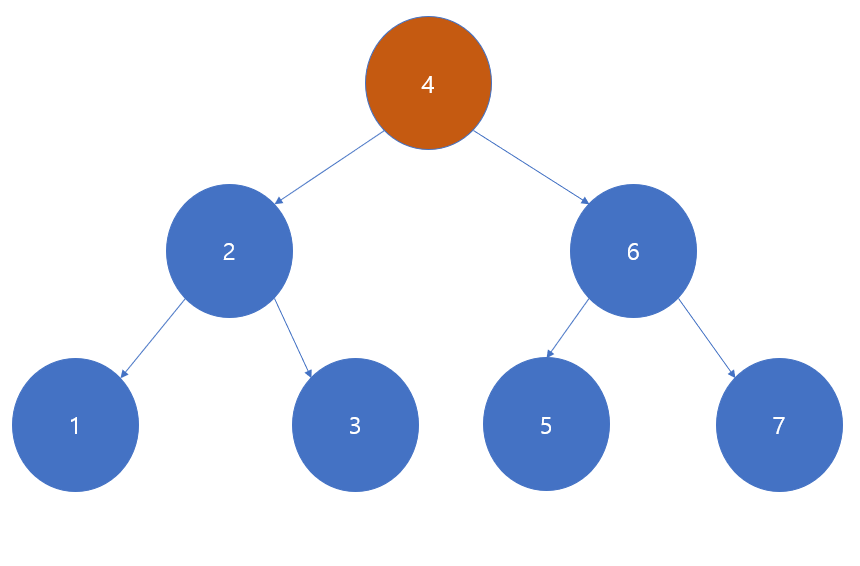
루트 4를 삭제한 경우

바로 앞 값 5을 올린다.

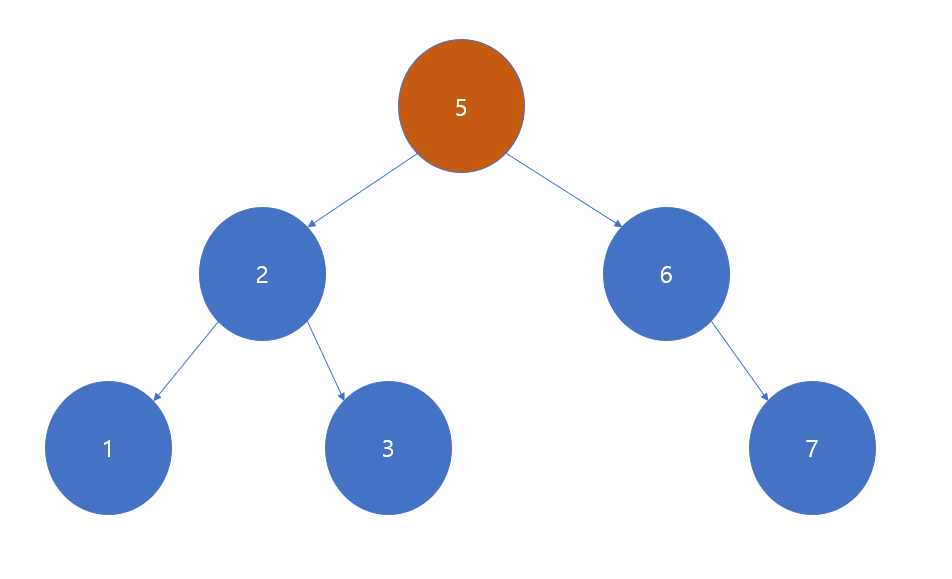
5는 리프이기 때문에 5자리를 지우고 루트자리에 5를 집어넣기만하면 된다.